The probability that the electron is in a shell of radius r and width
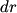 about the nucleus is about the nucleus is To find the maximum value of this probability, we find the zeroes of the derivative of 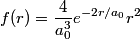 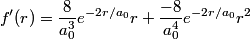 So, 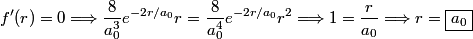 To check that this is a maximum, we compute that second derivative of 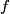 , ,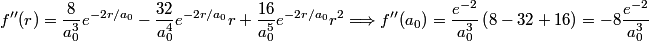 The negative second derivative implies that 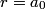 is a maximum. Therefore, answer (D) is correct. is a maximum. Therefore, answer (D) is correct. |